Math Symbols List
Contents
Math is a universal language used all around the world. For students learning English, understanding basic math symbols and expanding their math vocabulary can unlock this powerful subject.
In this article, we’ll explore several common symbols and their meanings to help you feel more confident in your math studies.
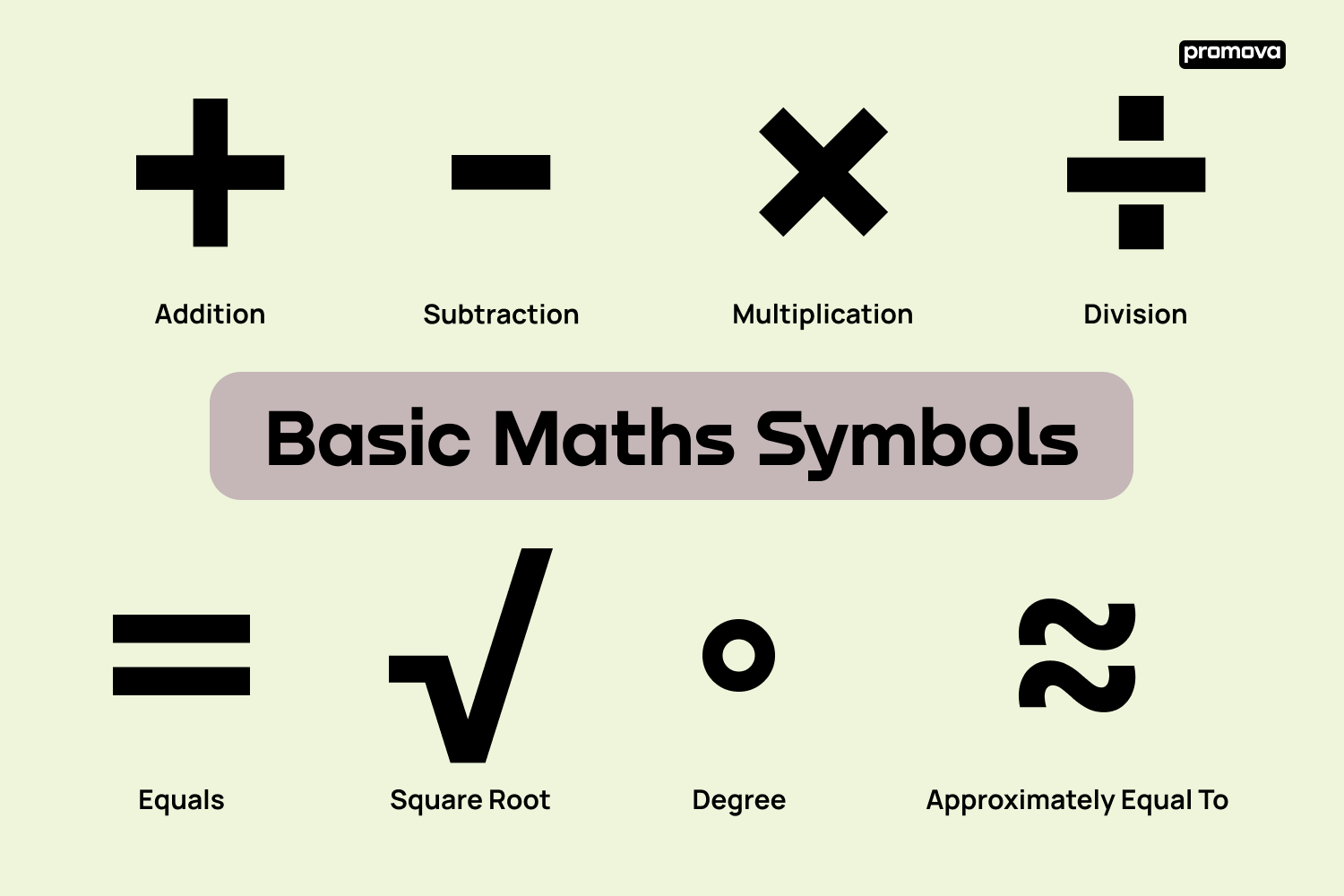
Basic Arithmetic Symbols
If you’re learning English, understanding the math symbols used in basic arithmetic is essential to make math communication more effective.
These are fundamental symbols that you’ll often see in everyday math problems:
| Symbol | Symbol Name | Math Operation | What is it used for? | Example |
| + | Plus | Addition | To add two or more numbers. | 5 + 3 = 8 |
| − | Minus | Subtraction | To subtract one number from another. | 9 − 6 = 3 |
| × | Times | Multiplication | To multiply numbers. | 4 × 2 = 8 |
| ÷ (/) | Divided by | Division | To divide one number by another. | 12 ÷ 4 = 3 |
By becoming familiar with these basic arithmetic symbols, you’ll take an important step toward understanding math expressions in English. This will help you a lot with daily tasks and your studies if you're learning math in English too!
Symbols in Algebra
Algebra often includes math symbols with names in English that can be a little confusing. However, these symbols are essential for forming and solving equations, representing unknowns, and expressing relationships.
Let’s explore them:
| Symbol | Name | Meaning | Example |
| x, y, z | x, y, z | Used to represent unknown numbers or variables. | y = 2x + 3 |
| = | Equals | Means two expressions are equal. | 5 = 5 |
| ≠ | Not equal | Shows two expressions are not equal. | 5 ≠ 6 |
| > | Greater than | Indicates one number is greater than another. | 7 > 3 |
| < | Less than | Indicates one number is smaller than another. | 2 < 9 |
| ≥ | Greater than or equal to | Shows one number is greater than or equal to another. | 5 ≥ 3 |
| ≤ | Less than or equal to | Shows one number is less than or equal to another. | 2 ≤ 3 |
| : | Ratio | Compares two quantities proportionally. | 1:2 (ratio of 4 to 8) |
| % | Percent | Refers to a part in a hundred. | 50% (half) |
Learning to recognize these algebraic symbols and their meanings will open doors for you to understand increasingly complex math expressions.
9
Symbols in Geometry
Geometry, with its unique shapes and relationships, has its own set of symbols that help describe shapes, angles, and spatial relationships.
It’s important to know how to refer to these symbols in English to communicate effectively:
| Symbol | Name | What is it? | Example |
| ∠ | Angle | The space between two lines that meet at a common point (vertex). | ∠ABC |
| ° | Degree | A unit of measure for angles. | 180° |
| ‖ | Parallel | Two lines that go in the same direction but never meet. | AB∥CD |
| ⊥ | Perpendicular | The intersection of two lines at a 90° angle. | AB⊥CD |
Mastering these geometry symbols will help you better understand spatial math and design concepts.
Advanced Math Symbols
Advanced math introduces symbols that may be completely new for some people because they are less common in everyday use. These are used in specialized areas like calculus, logic, and complex number theory:
| Symbol | Name | What is it used for? |
| ∪ | Union | To represent the combination of two sets; includes all unique elements from both sets. |
| ∩ | Intersection | To show common elements between two sets; includes only the elements in both sets.
|
| ∈ | Element of | To state that an object belongs to a set. |
| ² | Squared | To indicate a number raised to the power of two. |
| ³ | Cubed | To indicate a number raised to the power of three. |
| √ | Square Root | To represent the value that, when squared, equals the original number. |
| sin | Sine | A trigonometric function showing the ratio of the opposite side to the hypotenuse in a right triangle. |
| cos | Cosine | A trigonometric function showing the ratio of the adjacent side to the hypotenuse in a right triangle. |
| ∞ | Infinity | To describe something without limits. |
| ≈ | Approximately equal to | To show that two numbers are almost, but not exactly, equal. |
| f(x) | Function | To represent a rule that assigns exactly one output to each input. |
| d/dx | Derivative | To indicate how a function changes when its input changes by a small amount. |
| ∫ | Integral | To represent the accumulation of quantities, used in calculus to find areas under curves. |
| ⇒ | Implies | To denote that one statement implies another; used mainly in logic. |
| ≡ | Equivalent | To show that two statements are logically equivalent. |
| ℙ | Prime | To describe numbers that have only two divisors (1 and themselves). |
| x̄ | Mean | To calculate the average of a set of numbers (sum of all values divided by the number of values). |
| σ | Standard Deviation | To measure how spread out the values in a set are. |
| T | Transpose | To switch the rows and columns in a matrix (used in linear algebra). |
| · | Dot Product | To represent the sum of the products of the corresponding entries in two sequences of numbers. |
| i | Imaginary Unit | To define numbers that are not real. |
| ≅ | Congruent | To describe figures that have the same size and shape; two triangles are congruent if their corresponding sides and angles are equal. |
| π | Pi | To represent the ratio of a circle’s circumference to its diameter, approximately 3.14159. |
| ∀ | For all | To denote that a statement applies to all members of a certain set. |
| ∃ | There exists | To specify that at least one element satisfies a particular property, used in logic and set theory. |
Understanding these advanced math symbols will be very useful if you decide to dive deeper into higher-level math studies.
Conclusion
Math symbols are essential for understanding and clearly expressing mathematical ideas. From the addition sign (+) to pi (π), these symbols act as a universal language that makes learning and problem-solving easier anywhere in the world.
At first, it may seem confusing, but with constant practice, using these symbols will become intuitive. Knowing their names in English will also give you an advantage in your studies or future career.
Keep practicing your new vocabulary and enjoy exploring the fascinating world of math!


Comments